Tutorial Week 6

Worksheet Questions

1. Salt water with concentration of 1 g/L flows into Tank A at a rate $a$ L/min. The mixed solution in Tank A flows into Tank B at a rate $b$ L/min. Another pipe takes the solution in Tank B back into Tank A at a rate $c$ L/min. Finally, solution drains out of Tank B at a rate $a$ L/min. Note: the volume in each tank is the same and $a$+$c$=$b$ (to ensure that the volumes in the tanks are constant).
   1. Write down the system that gives the amount of salt in each tank at any given time.
   2. Show that it is impossible for this system to have oscillations (damped or otherwise).
2. Plot the phase plane for the following systems of equations. Your phase plane should include eigen-directions for real eigenvalues and several solutions illustrating the general shapes of solutions in the phase plane.
   1. \begin{eqnarray} x_1' &=& x_1 -8x_2 \\ x_2' &=& 8x_1+x_2 \end{eqnarray}
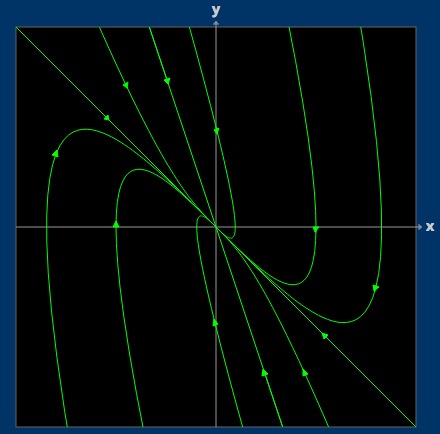
   2. \begin{eqnarray} x_1' &=& x_2 \\ x_2' &=& -3x_1-4x_2 \end{eqnarray}

Solutions:

1. (6pts+2pts)
   1. \begin{eqnarray} Q_1^\prime &=& (1)\cdot a -b\frac{Q_1}{V} + c\frac{Q_2}{V} \\ Q_2^\prime &=& b\frac{Q_1}{V} -(a+c)\frac{Q_2}{V} \end{eqnarray} For each equation, 1 pt for form (positive and negative terns in a DE), 2 pts for the details of the terms (Q, V) so total 6 pts.
   2. Eigenvalues of the homogeneous equation are: $-b \pm \sqrt{bc}$ (1 pt). Since b,c $\ge$ 0, the eigenvalues are real and the system can not have oscillations (1 pt - must state this explicitly).
2. (3pts+6pts)
   1. 1 pt for eigenvalues (1+ 8i & 1-8i) , 1 pt for growing spiral, 1 pt for direction of rotation (must have some justification for choice)
   2. 1 pt for eigenvalues (-1& -3), 2 pts for eigenvectors (e.g. (-1,1) for -1, and (-1, 3) for -3), 3 pts for drawing (1 pt for including a solution in each of the two eigen-directions with arrows correct, 1 pt for shape of non-eigen solutions, 1 pt for direction of arrows on non-eigen solutions).

Here are example answers to these two questions. The vector fields are not necessary but there should be arrows showing direction along the eigen-directions and the solution curves.

https://www.desmos.com/calculator/oy3oslqfya
https://www.desmos.com/calculator/60djgxc0hn (Drag the blue point to see different solution curves.)