Exam review questions

1. How many distinct possibilities are there for $\lim_{t\to\infty}x(t)$ where $x(t)$ solves the equation $x'-x=e^t$?
   1. 1
   2. 2
   3. 3
   4. 4









2. Find the solution to the equation $y'=-x/y$ with the initial condition $y(0)=-2$.
   1. $y=\sqrt{-4-x^2}$
   2. $y=\sqrt{4-x^2}$
   3. $y=-\sqrt{4-x^2}$
   4. $x^2+y^2=4$









3. To solve the equation $y''+16\pi^2 y=f(t)$ using the Method of Undetermined Coefficients where $f(t)$ is a periodic function with period $T$ and $2T$ is not an integer, your guess at the particular solution should be
   1. $y_p(t)=A \sin(4 \pi t) + B \cos(4\pi t)$
   2. $y_p(t)=A_0 + \sum_{n=1}^\infty a_n\cos\left(\frac{n \pi}{T} t\right) + \sum_{n=1}^\infty b_n\sin\left(\frac{n \pi}{T} t\right)$
   3. $y_p(t)=A_0 + \sum_{n=1}^\infty a_n\cos\left(\frac{2n \pi}{T} t\right) + \sum_{n=1}^\infty b_n\sin\left(\frac{2n \pi}{T} t\right)$
   4. $y_p(t)=A_0 + \sum_{n=1}^\infty a_n\cos\left(\frac{2n \pi}{T} t\right)$









4. The solution to the equation $y''+81y=f(t)$ where $f(t)$ is a switching function that continually goes on ($f(t)=1$) for one second and off ($f(t)=0$) for 1 second repeatedly. The solution consists of many sinusoidal functions but the one with largest amplitude has a period of
   1. $\omega = \pi$
   2. $\omega = 2\pi$
   3. $\omega = 3\pi$
   4. $\omega = 4\pi$









5. Consider the matrix equation $x'=Ax$ where $A=\begin{pmatrix} a & 3 \\ 1 & a \end{pmatrix}$. As the value of $a$ increases smoothly from a large negative number to a large positive number, the classification of the steady state...
   1. ... changes from a stable node to a stable spiral to an unstable spiral to an unstable node.
   2. ... is always a saddle.
   3. ... changes from a stable node to a saddle to an unstable node.
   4. ... changes from an unstable node to a saddle to a stable node.









6. The inverse transform of the function $\displaystyle Y(s)=\frac{s+3}{(s+1)^2+4}$ is
   1. $\sqrt{2}e^{-t}\cos\left(2\left(t-\frac{\pi}{8}\right)\right)$
   2. $\sqrt{2}e^{-t}\cos\left(2\left(t-\frac{\pi}{4}\right)\right)$
   3. $\sqrt{2}e^{-t}\sin\left(2\left(t-\frac{\pi}{8}\right)\right)$
   4. $\sqrt{2}e^{-t}\cos\left(2\left(t+\frac{\pi}{4}\right)\right)$









7. Which of the following are the eigenfunctions for the diffusion equation $u_t=16u_{xx}$ with boundary conditions $u(0,t)=0$ and $u_x(4,t)=0$?
   1. $\displaystyle u_n(x,t)=e^{-\frac{n^2\pi^2}{4}t} \sin\left( \frac{n\pi x}{8} \right)$ for $n$ even.
   2. $\displaystyle u_n(x,t)=e^{-n^2\pi^2t} \sin\left( \frac{n\pi x}{4} \right)$ for $n$ even.
   3. $\displaystyle u_n(x,t)=e^{-\frac{n^2\pi^2}{4}t} \sin\left( \frac{n\pi x}{8} \right)$ for $n$ odd.
   4. $\displaystyle u_n(x,t)=e^{-n^2\pi^2t} \sin\left( \frac{n\pi x}{4} \right)$ for $n$ odd.









8. Which of the following functions would be the correct choice for extending $f(x)$ in order to solve the diffusion equation on the interval $[0,1]$ with initial condition $f(x)=x$ and boundary conditions $u_x(0,t)=0$ and $u(1,t)=0$?
   (a)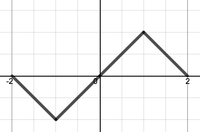 . . (b)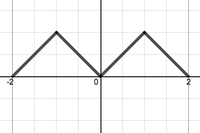
   
   (c) . . (d)









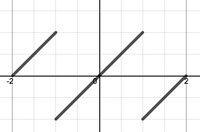
9. You add 5 grams of salt per minute through the left end ($x=0$) of a 3m pipe filled with water. The salt diffuses in the pipe with diffusion coefficient $D=2$ m$^2/$min. The right end of the pipe ($x=3$) is connected to an extremely large tank that starts with no salt in it. What is the steady state concentration profile through the pipe?
   1. $c_{ss}(x) = \frac{5}{2}x-\frac{15}{2}$
   2. $c_{ss}(x) = -\frac{2}{5}x$
   3. $c_{ss}(x) = -\frac{2}{5}x+\frac{6}{5}$
   4. $c_{ss}(x) = -\frac{5}{2}x+\frac{15}{2}$









10. Choose the best option for a guess at the particular solution to the equation $y'''+2y'' = t + e^{-2t}$.
    1. $ y_p(t)=At+B+Cte^{-2t}$
    2. $ y_p(t)=At^2+Bt+Cte^{-2t}$
    3. $ y_p(t)=At^2+Bt+Ce^{-2t}$
    4. $ y_p(t)=At^3+Bt^2+Cte^{-2t}$









11. Books are taken out of a library at a constant rate. Books are returned to the library at a rate proportional to how many books are currently checked out from the library. On the 8th day of the semester, a large delivery of new books arrives at the library. Choose the best model for the number of books in the library $B(t)$ at time $t$ measured in days from the start of the semester.
    1. $\frac{dB}{dt} = r(B_0-B) - c + a\delta(t-8)$
    2. $\frac{dB}{dt} = r(B_0-B) - c + au_8(t)$
    3. $\frac{dB}{dt} = c - rB + a\delta(t-8)$
    4. $\frac{dB}{dt} = c - rB + au_8(t)$









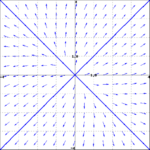
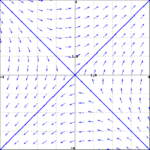
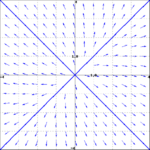
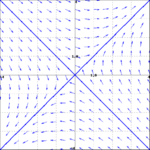
12. Match the general solution to the vector field
    1. $\mathbf{y}(t) = c_1 e^{8t}\begin{pmatrix} 1 \\ 1 \end{pmatrix}+c_2 e^{4t}\begin{pmatrix} 1 \\ -1 \end{pmatrix}$
    2. $\mathbf{y}(t) = c_1 e^{8t}\begin{pmatrix} 1 \\ 1 \end{pmatrix}+c_2 e^{-4t}\begin{pmatrix} 1 \\ -1 \end{pmatrix}$

    (i) (ii) (iii) (iv)

    1. (i) -- A; (ii) -- B.
    2. (i) -- C; (ii) -- B.
    3. (i) -- A; (ii) -- D.
    4. (i) -- C; (ii) -- D.